EMF Equation Of Transformer

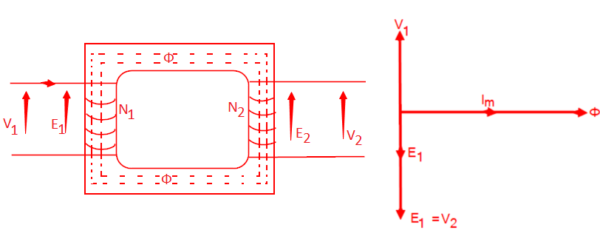
⇒ Let us consider an ideal transformer on no load, when an alternating voltage V₁ is applied to the primary side of the transformer thus magnetizing current start flowing in the primary winding and flux Φ is generated in the transformer core.

⇒ The flux Φ is uniformly distributed around the transformer core and it is linked with both primary and secondary winding of the transformer.

⇒ The flux Φ is alternating in nature therefore according to Faraday’s law of electromagnetic induction, emf is induced in the primary side or winding of the transformer.

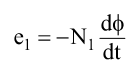
⇒ According to Lenz’s law, the induced emf e₁ is equal and opposite the supply voltage V₁

⇒ Since the source given to the primary is sinusoidal therefore the flux produced is also sinusoidal in nature. Hence the sinusoidal flux Φ produced by the primary can be represented as.
Φ = Φ_m sin ωt

⇒ Putting the value of Φ we get

⇒ On differentiating the above equation we get

e₁ = -ω N₁ Φ_m cosωt

e₁ = -2πf N₁ Φ_m cosωt ……(since ω = 2πf )

e₁ = 2πf N₁ Φ_m sin(ωt – 90^.) . . . . . (1)

⇒ From equation 1 it is clear that the induced emf lags flux by 90 degree hence maximum value of induced emf will be
e_max = 2πf N₁ Φ_m

⇒ The rms value can be obtained by dividing the maximum value of induced emf by √2 therefore

Note: From equation 1 it is clear that induced emf e₁ in the primary winding lags behind the flux by 90^o. Similarly, the induced emf e₂ in the secondary lags behind the flux by 90^o

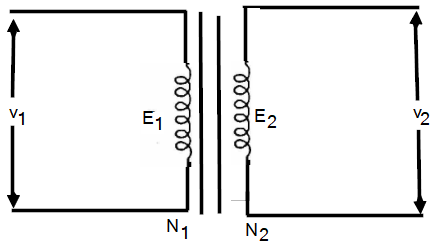
Voltage Transformation Ratio (K)

The constant K is called as Voltage transformation ratio and it is given as:

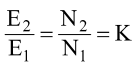
For an ideal transformer, there is no voltage drop in the windings, therefore, E₁ = E₂ and V₁ = V₂.

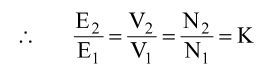
If N₂ > N₁ and K >1 then the transformer is called as step up transformer.
If N₁ > N₂ and K< 2 then the transformer is called as step down transformer.

There are no losses in an ideal transformer therefore volt-ampere input of the primary will be always equal to the volt ampere of the secondary.

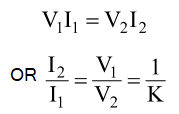
From above equation, it is clear that current is inversely proportional to the voltage i.e if we will increase the value of voltage than the value of current will decrease.